The Hidden Math in Everyday Life: Dr. Karl-Dieter Crisman’s Research on Cyclic Orders  

When Election Day arrives in just a few months, millions of Americans will line up at voting booths and cast their ballots for a presidential candidate. Each vote counts once toward the final popular vote, or one point.  

But what if Americans could give votes to six candidates, and their favorite got six points and their least favorite only got one? If people had the option to rank up to six candidates instead of picking just one, there’s 720 possible voting combinations. Even with close to a thousand voters, each option could come up just once or twice. And not every option for completing a process is efficient or harmonious. What if there was a way to analyze all the possible ways to vote mathematically? 

Dr. Karl-Dieter Crisman (mathematics and computer science), along with former research students Abraham Holleran ’22, Micah Martin ’21 and Josephine Noonan ’23, asked questions like these while conducting research about voting on cyclic orders from 2020 to 2023. They recently published a research article in “Mathematical Analyses of Decisions, Voting and Games,” Volume 795, of Contemporary Mathematics, a well-known series of conference proceedings in academic math. 

What are Cyclic Orders? 

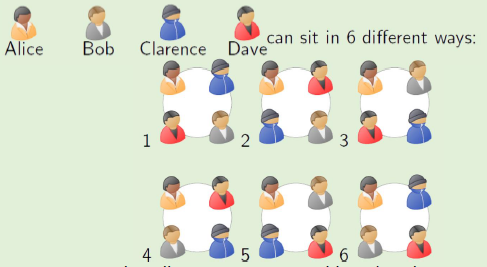
Cyclic orders, simply put, are ways to arrange a set of objects or people in a circle, and they’re present in many areas of our lives. For example, when setting the Thanksgiving table, you may want to place certain people, like couples, together. But there may be others who need to sit apart. How do you decide who should sit where? Should one person decide, or should everyone get a vote? Can they only vote for who they want to sit next to, or also for who they don’t want to sit by? 

While applications could include the order of job reviews or Thanksgiving seating, a good real-life example is poker. “I don’t condone gambling, of course,” says Crisman. “But it’s possible to bet on the winner of the World Series of Poker, and for that it matters where your favorite player sits. If they’re sitting right after a strong player, that’s bad. But sitting right before a strong player is good. If in that betting people got to vote on who sits where, how much money would people be willing to give or donate to ensure the player they want to win is sitting in the best spot? And how would they calculate it?” 

Investigating the Math 

The research started with making manual drawings of figures around tables and mentally calculating the different options for a seating arrangement that gives the participants the chance to vote on who sits where. They used concepts commonly used in math, like symmetry, one-to-one relationships and induction.  

Later, Holleran (a computer science double major) coded a program that would calculate all the possible seating options depending on the preferences of the people voting for who sits where. Once the students created a seemingly working system, they then spent the rest of their time trying to break the system in order to perfect it.  

“We found its strengths and weaknesses and any unexpected results that can arise. For instance, we were looking for times when all the people voting want a certain aspect of the seating arrangement, but because of how the system is set up that configuration is not in the seating arrangement that wins,” said Noonan.  

Why is Mathematics Important? 

The students finally found a good solution for what happens with different systems with four or five people at a table. The students went on to present their findings at several virtual conferences, including the Joint Mathematics Meetings—the world’s largest conference of mathematics—which led to publication in the Contemporary Mathematics volume.  

“The reason why it’s cool is that it’s undergraduates publishing in a math journal. It’s much more typical for that to happen in experimental sciences,” said Crisman. “It’s a lot harder in the mathematical sciences—math papers have to have a high level of logical rigor, higher than most academic fields. It has to be interesting, but it also has to be math. Putting it all together with the logic and the reasoning and the proof made it publishable.” 

“Math is just another way that the God of Creation is asking us to be fully human.” 

Dr. Karl-Dieter Crisman

The research was valuable academically and practically for each student. “When someone says ‘I’m a mathematician,’ most people don’t know what that looks like,” Holleran said. “But the mathematical skills of critical thinking and quantitative thinking skills…will serve you no matter where you go. Lots of life’s problems—at your job, in your hobbies, in your relationships—can be understood better by applying mathematical problem-solving.”  

Crisman hopes that hands-on research experiences like this will inspire more students to study and research math at Gordon. “You might not think faith is connected to math, but this research shows there’s actually a lot of compassion and truth, and very interesting philosophical, even theological content in how cyclic orders play out. Another outcome might be justice—what is a just way to allocate resources? Math is just another way that the God of Creation is asking us to be fully human.”